System Of Linear Differential Equations
System of linear differential equations. Because they involve functions and their derivatives each of these linear equations is itself a differential equation. This will include deriving a second linearly independent solution that we will. As an example we show in Figure 51 the case a 0 b 1 c 1 d 0.
Beginaligned fracmathrmdRmathrmdt aR5em fracmathrmdJmathrmdt dJ enspace. Two unknowns and two equations suggests the elimination method from algebra. To address such problems this paper proposes a general method of using a memristor-capacitor M-C circuit to solve inhomogeneous linear ODEs and.
We start our analysis with a silly example that will nevertheless help up understand how higher dimensional systems can exhibit new and interesting dynamics that we do not see in one dimension. Due to the coupling we have to connect the outputs from the integrators to the inputs. The general form is xt axtbyt yt cxtdyt 2 with x0 x.
The system is nongeneric that is the determinant of the matrix of coefficients is zero. X 1 x1 2x2 x 2 3x12x2 x 1 x 1 2 x 2 x 2 3 x 1 2 x 2. Example The linear system x0.
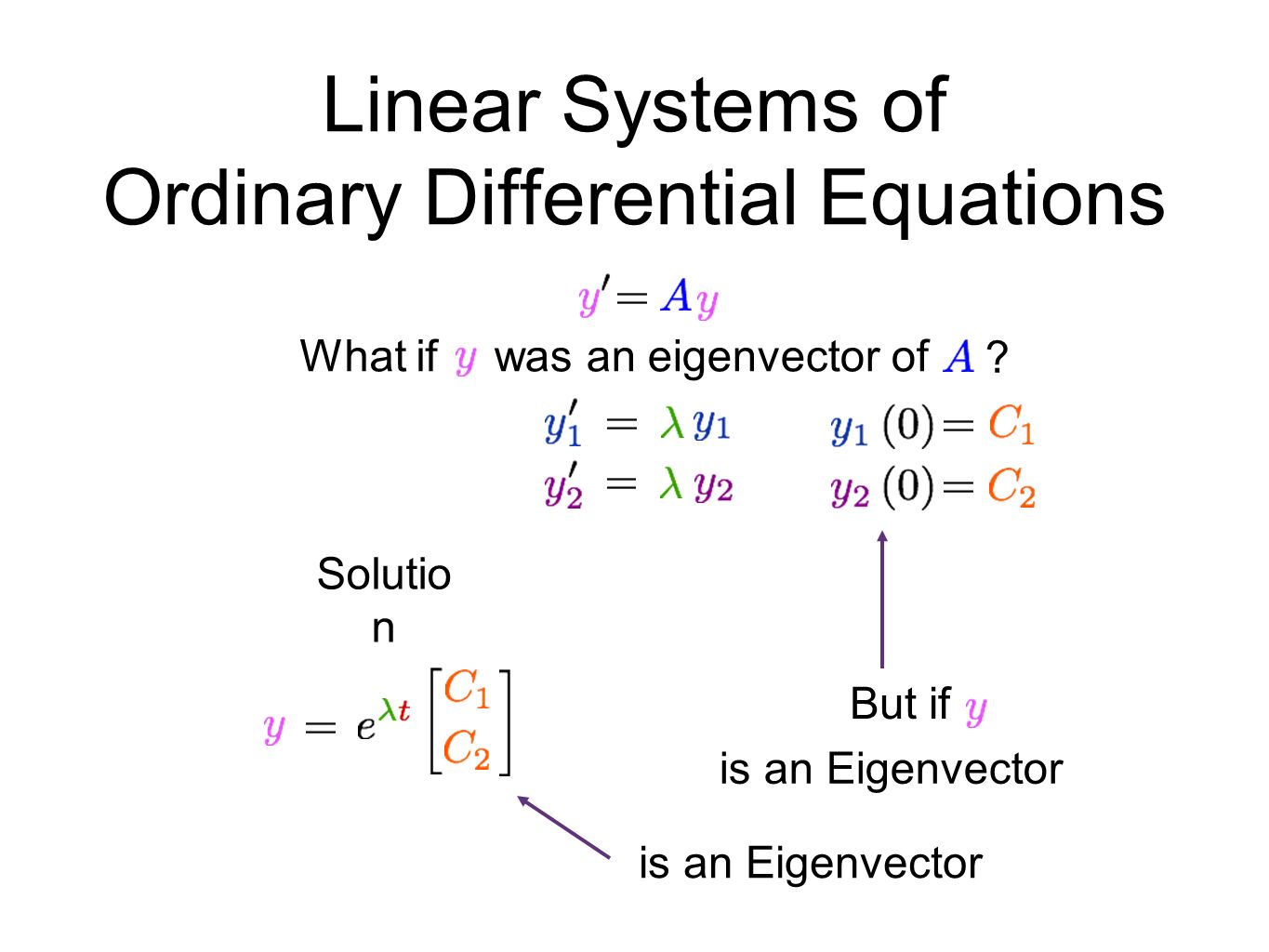
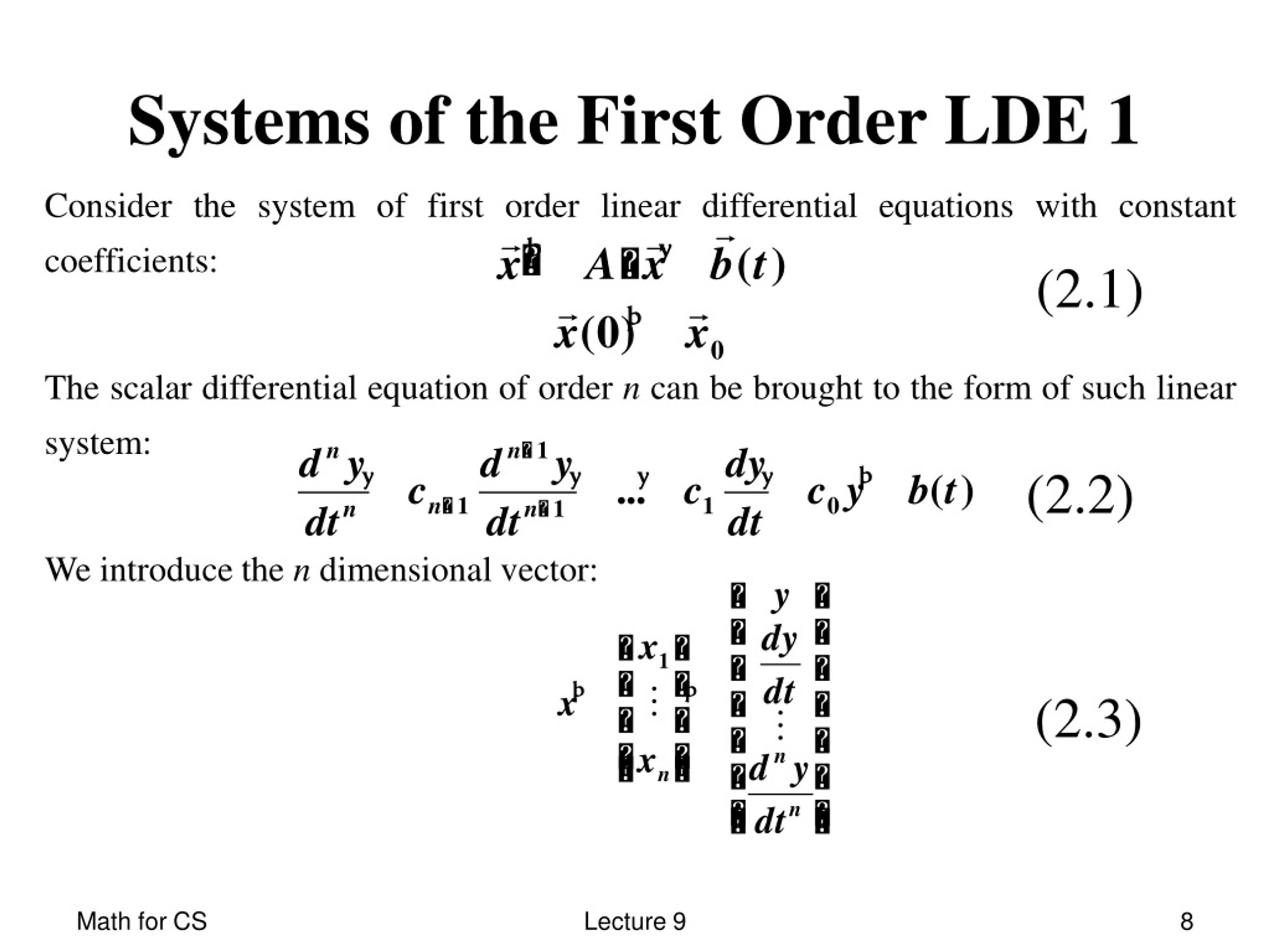
Syms u t v t Define the equations using and represent differentiation using the diff function. Systems of Differential Equations 51 Linear Systems We consider the linear system x0 ax by y0 cx dy51 This can be modeled using two integrators one for each equation. A linear homogeneous system of differential equations is a system of the form beginaligned dot x_1 vdots dot x_n a_n1x_1 cdots a_nnx_n endaligned which we can abbreviate in matrix form to dotvx Avx where vx beginbmatrix x_1vdotsx_n endbmatrix qquad A.
In this video you will learn about differential equations and how linear algebra can help in solving differential equations. Du dt 3 u 4 v dv dt - 4 u 3 v. Here is an example of a system of first order linear differential equations.
We call this kind of system a coupled system since knowledge of x2 x 2 is required in order to find x1 x 1 and likewise knowledge of x1. Real Eigenvalues Solving systems of differential equations with real eigenvalues.
Imagine a distant part of the country where the life form is a type of cattle well call the xnay beast that eats a certain type of grass well call.
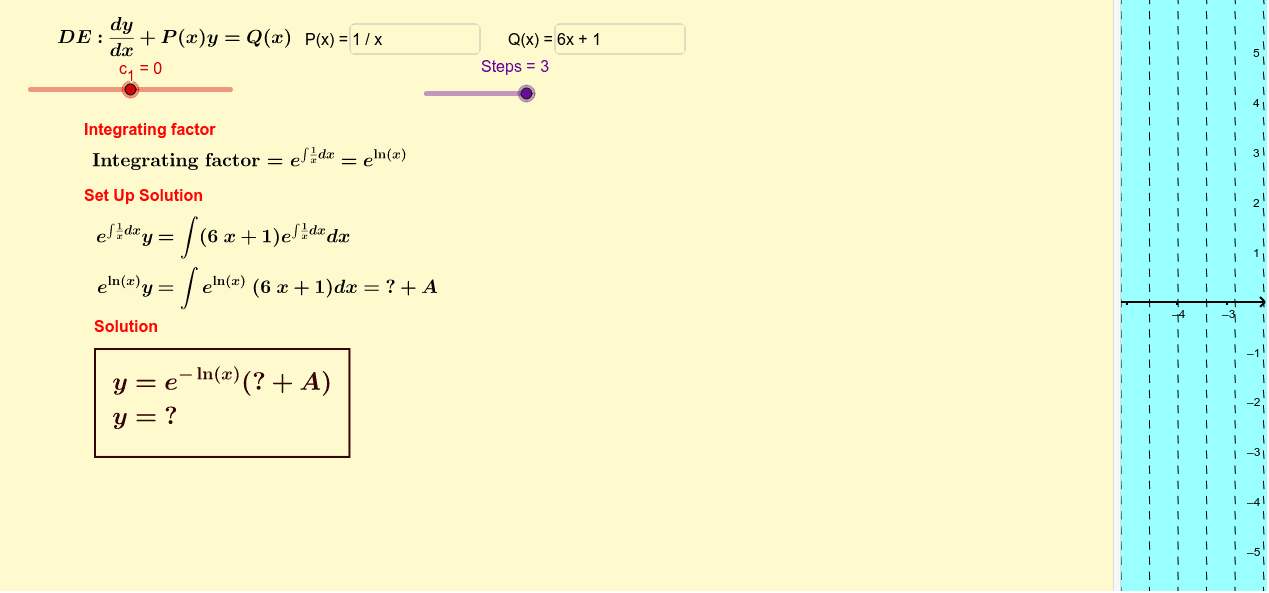
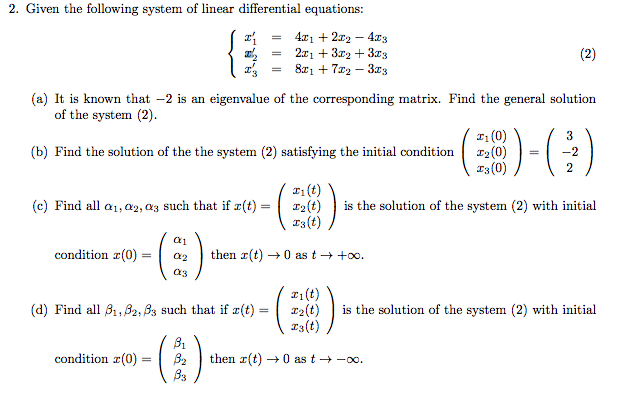
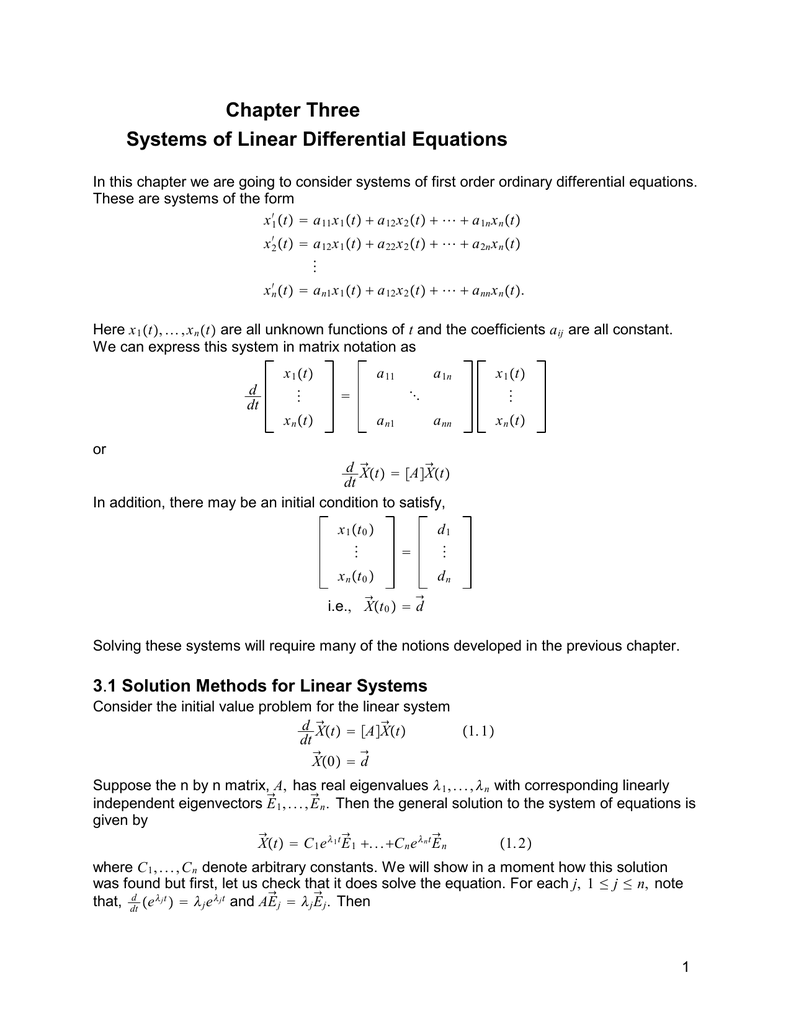
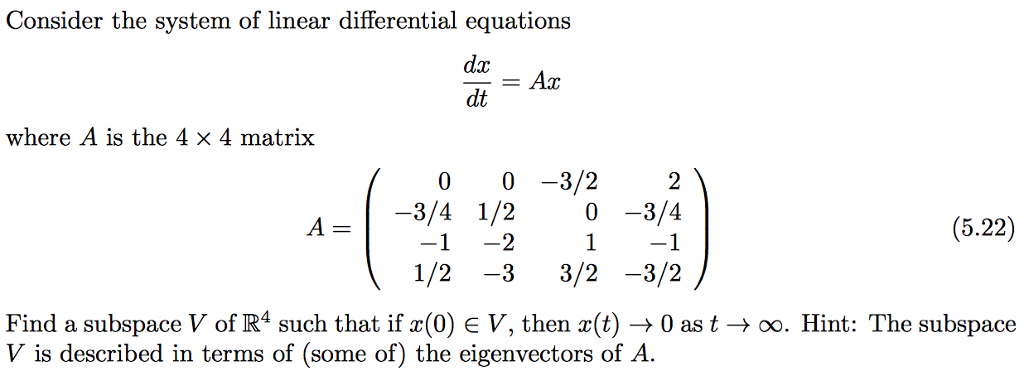
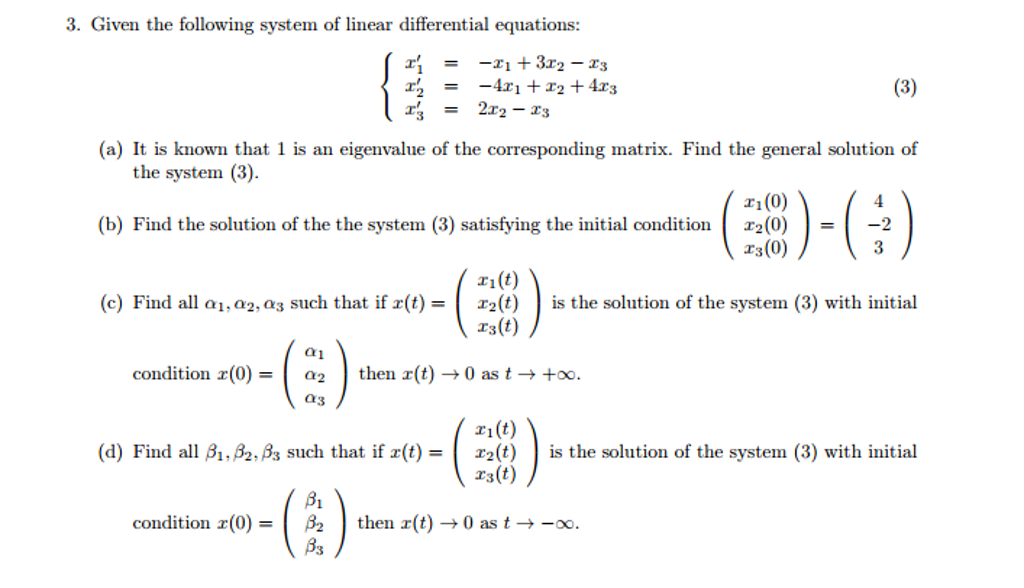
System of differential equations. First represent u and v by using syms to create the symbolic functions u t and v t. Thus our hypothetical coupled system of linear differential equations is. Endaligned Using the results from above the solutions to the two differential equations are. Here is an example of a system of first order linear differential equations. A system of linear differential equations is a set of linear equations relating a group of functions to their derivatives. Equations Math 240 First order linear systems Solutions Beyond rst order systems First order linear systems De nition A rst order system of di erential equations is of the form x0t Atxtbt. Consider the system of linear differential equations. A system of n linear first order differential equations in n unknowns an n n system of linear equations has the general form.
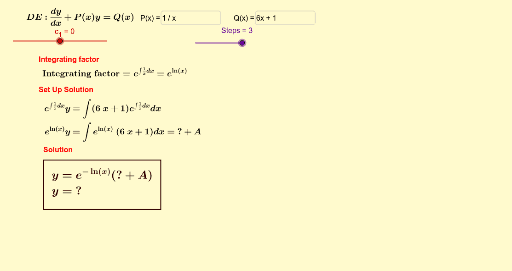
Imagine a distant part of the country where the life form is a type of cattle well call the xnay beast that eats a certain type of grass well call. Inhomogeneous linear ordinary differential equations ODEs and systems of ODEs can be solved in a variety of ways. X0 nt an1tx1tan2tx2t anntxntf1t. A linear homogeneous system of differential equations is a system of the form beginaligned dot x_1 vdots dot x_n a_n1x_1 cdots a_nnx_n endaligned which we can abbreviate in matrix form to dotvx Avx where vx beginbmatrix x_1vdotsx_n endbmatrix qquad A. 0and y0 y. Here is an example of a system of first order linear differential equations. We start our analysis with a silly example that will nevertheless help up understand how higher dimensional systems can exhibit new and interesting dynamics that we do not see in one dimension.





















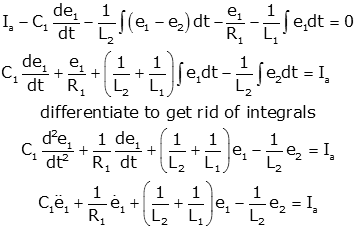






Post a Comment for "System Of Linear Differential Equations"